What Is Margin Of Error?
The margin of error (MOE)is a term that comes up frequently in market research reports and even in casual conversation. So, what exactly does it imply, and how does it relate to your survey data?
A margin of error measures accuracy or precision and suggests how close a survey result is to the actual population value. The margin of error is a statistic expressing the sampling variability of an estimate obtained from a poll or survey. The larger the margin of error, the less confident we are in the accuracy of the forecast.
How Does MOE Work?
Let's assume your task is to perform a survey of employees at your organization to determine whether they would prefer a bonus in cash or PTO (personal time off).
Imagine you have the tall task of surveying 10,000 employees. Instead of spending time and resources probing all 10,000 employees, it's better to gather a sample and assume that if we use a cross-section of the whole population, it will provide an accurate approximation.
The issue here is that it's challenging to select a sample group that mirrors the entire employee population and includes the extremes.
The acceptable margin of error is generally between 4% and 8% at the 95% confidence level. Getting a precisely representative sample, on the other hand, is the real trick.
When conducting a survey, bear in mind that you are attempting to represent a larger group with a smaller number of individuals. Consider the term MOE as a method for determining how effective your survey is and how accurately the sample group represents the larger population. A smaller margin of error means you can have confidence in your results. Alternatively, a considerable margin might suggest that the opinions of the sample group deviate from the views of the total population.
So how do we find the balance?

Sample Size Matters
The sample size is critical for obtaining accurate, statistically significant results and running your survey successfully. The right balance yields valid results but is not so large that it increases the cost and time required to conduct the survey.
A sample group that is too small, whether a random selection or a more intuitive approach like stratification, may omit data. This can produce anomalies in the sample that skew the findings and inaccurately represent the whole population.
The size of the sample affects both sampling and non-sampling error. The larger the sample, the less sampling error there will be. However, non-sampling error will increase with sample size because there are more opportunities for things to go wrong.
If the sample is too large, the survey might become costly and time-consuming. Although findings will be more accurate, they don't compensate for the added strain on resources. The right sample size for your survey depends on the sort of data you need to collect and the level of precision you need.
Generally, you want a sample size that will produce results with a margin of error no larger than 5%, meaning that 95% of the time, the results from your survey will be within 5% of the true population value.
How To Find Margin Of Error
The margin of error is the amount by which you want to deviate from the total population of respondents in order to obtain a specific result. So its significance goes a long way in determining how accurate your survey is.
Here’s the formula:
Find the population standard deviation (σ) and sample size (n).
Divide the square root of your sample size into your population standard deviation
Multiply the product by the standard score.
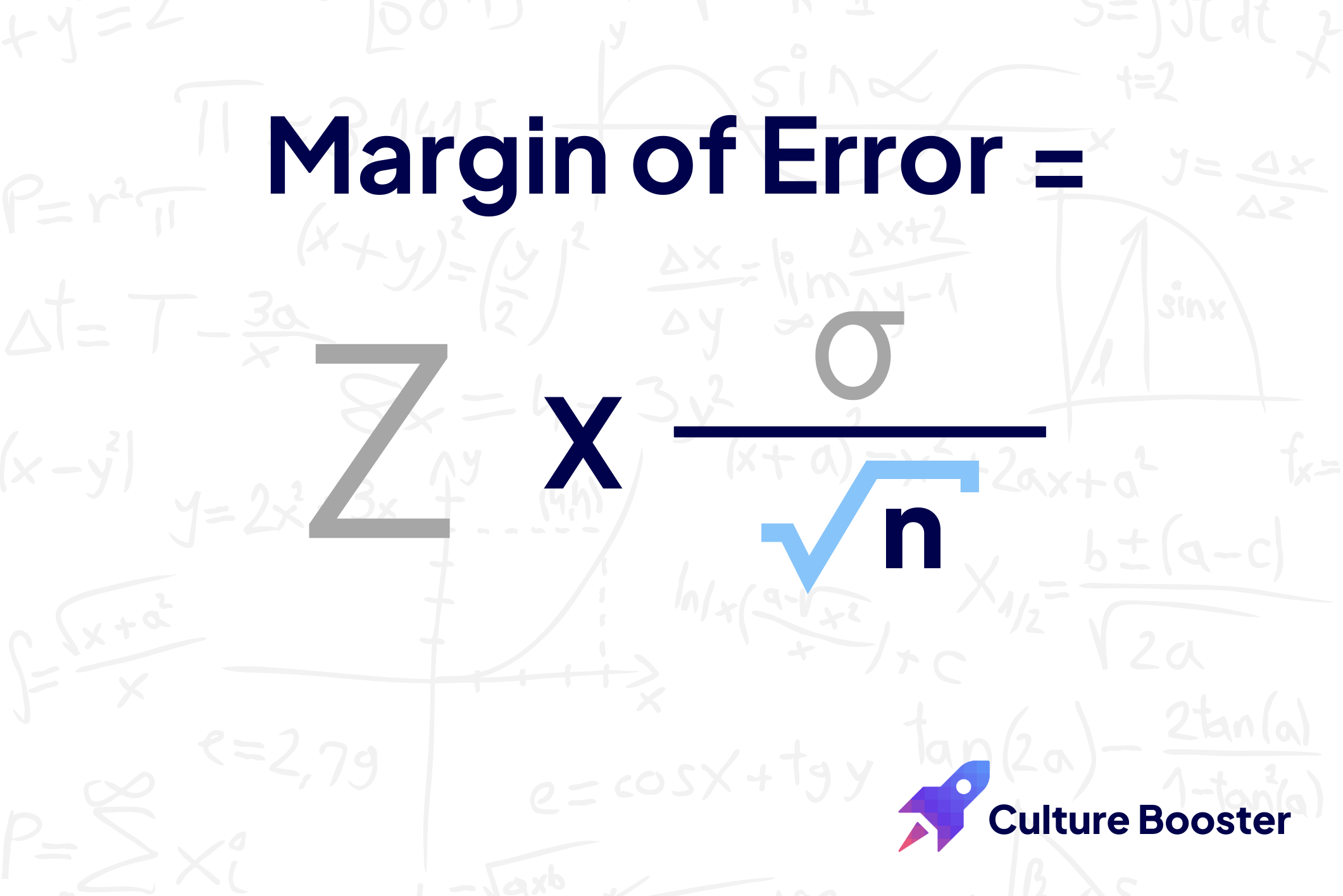
Key:
n = sample size
σ = population standard deviation
z = standard score
Still not sure how to calculate margin of error? That’s okay, we get it.
Margin Of Error Calculator
Accuracy is key when it comes to data. With our margin of error calculator tool, you can rest assured that your numbers are as accurate as possible. This will help you make better decisions for your business and reach your goals faster.
If you’re concerned human error will damage the reliability of your results, Culture Booster has your back!
For the most accurate margin of error calculation, check out:
Culture Booster’s Free Margin Of Error Calculator
Decrease Margin Of Error With This 4 Point Plan
Reducing the margin of error is an essential component of increasing the dependability of your surveys and getting better, more accurate results.
- Increase your sample size: Increasing your sample size is the simplest method to reduce the margin of error. Having a larger number of people complete your surveys statistically ensures a more accurate representation of the population polled. Increasing the sample size also helps to minimize the effects of individual variability.
- Reduce confidence level: A lower confidence level indicates a more accurate error margin—but don't be fooled by this because lowering the confidence level reduces the survey's certainty that the results are accurate.
- Decrease the number of variables: It's a good idea to limit the variables in your study. The more variables you have, the more likely you will introduce an error into your results. By reducing the number of variables, you can minimize this potential for error.
- Use a reliable sampling method: A second way to reduce the margin of error is to use a reliable sampling method. Select your sample group carefully to ensure that it is representative of the population you're studying. If you don't use a reliable sampling method, your results may be skewed, and the margin of error will be higher.

Margin Of Error Glossary
It's hard enough to calculate and understand statistics without having to wade through all of the related terms. We want to make things easier for you.
Here are some helpful glossary notes to ensure you get the best insight by relying on accurate results!
Sampling Errors Vs. Non-Sampling Errors
Statistics and surveys are practical approximations used to examine populations. Statistics are not exact; there are variables to bear in mind. These variables are referred to as errors.
There are two key MOE factors that determine the accuracy of survey data:
Sampling errors or “standard errors” occur when a sample does not represent the population it is drawn from. These kinds of errors are generally due to an inclusion error, which means your sampling frame does not include the people you wish to analyze.
Non-sampling errors encompass all the other factors that can affect survey data.
Including:
- Interviewer bias: This is when interviewers consciously or unconsciously give respondents different answers or rate them differently based on their personal biases.
- Poor questionnaire design: This can lead to inaccurate responses from respondents, as they may not understand the questions or be confused about what is being asked.
- Non-response error: AKA non-response bias, occurs when members of a sample group do not respond to the census or do not answer questions truthfully or accurately.
All of these factors can have a significant impact on the results of a survey, and it is important to be aware of them when interpreting survey data.
The margin of error is the radius of a confidence level for a given statistic. For total transparency, it's good to report MOE when presenting survey results or statistics.
Understanding Confidence Level And Standard Deviation
When conducting surveys, it's essential to understand confidence level and standard deviations, their relation to MOE, and how they're used.

Confidence Level Vs Confidence Interval
The two concepts are related, but they are not the same. The confidence level tells you how sure you can be in the estimate, while the confidence interval tells you what range of values is likely to include the true value.
True Value: “The actual population value that would be obtained with perfect measuring instruments and without committing any error of any type, both in collecting the primary data and carrying out mathematical operations”. Glossary Of Statistical Data Terms
The margin of error is the maximum amount by which an estimate can be wrong and still be considered accurate. The confidence interval is a range of values likely to contain the true value. It is calculated based on the sample size and the variability of the data. The higher the confidence level, the narrower the interval will be.
For example, suppose you want to know how many people will vote in an election. You can poll a small number of people and get an estimate, but there will always be some uncertainty about whether that estimate is accurate for the whole population. The confidence level tells you how confident you can be that your assessment represents the population as a whole.
Generally speaking, confidence intervals are wider than margins of error because they reflect sampling error and variability in the estimate due to factors such as population size. In contrast, the margin of error quantifies only the sampling errors.
Confidence level and confidence interval are two ways of thinking about the uncertainty in a statistical estimate. The confidence level is the probability that the true value falls within the confidence interval.
Standard Deviation
Standard deviation measures the spread of data points from the mean (average) value. It's calculated as the square root of the variance, which is the sum of squared deviations from the mean.
Variance is clustered around the mean results in a small standard deviation, and we can be reasonably confident in our measurements. If the standard deviation is significant, then our data is more spread out, and we should be less confident in the accuracy of our results.
For example, imagine that you ask 100 people how tall they are. The average height might be 5 feet, 8 inches, with some people being taller and some being shorter. The standard deviation would show how much variation there is in the heights of the people you surveyed.
Understanding standard deviation is necessary because it gives us a way to measure the margin of error in our data set. This is especially important when dealing with data used to make decisions in business, politics, and medicine.
Standard Score Vs. Standard Deviation
A standard score is a way of measuring how far a particular score is from the mean. The standard deviation measures how spread out values are, and the standard score tells you how many deviations away from the norm a particular value is. In other words, it's a way of quantifying how unusual or extreme a specific result is.
Standard deviation is a measure of variability, or how spread out a set of data points is. It's calculated by taking the square root of the average of the squared differences between each data point and the mean.
A standard score is a way of measuring how far a particular score is from the mean. It's calculated by subtracting the mean from the score and dividing it by the standard deviation.
For example, if someone has a standard score of 2, they're two standard deviations away from the mean on the positive side. This would be pretty far out from the average, indicating that this person has an unusually high score. Alternatively, if someone has a standard score of -2, that would mean they're two standard deviations away from the mean on the negative side, indicating that their score is below average.
Key Takeaways
Survey accuracy is all about balancing sample sizes, the margin of error, and confidence level to obtain an acceptable conclusion with minimal resources.
Sample size is key to survey accuracy. The larger the sample size, the more accurate a survey will be. A more extensive sample gives you a greater chance of obtaining a representative result. However, getting a large sample size is not always practical or possible. In these cases, you can improve the accuracy of your survey by using a smaller margin of error and a higher confidence level.
A margin of error is the amount of uncertainty associated with a survey result. It tells you how confident you can be in the accuracy of the result. A smaller margin of error means that your survey is more accurate.
A confidence level is the percentage of times a survey will produce the same result if repeated. It tells you how confident you can be in the accuracy of the result. A higher confidence level means that you are more confident in the accuracy of the result.
When you combine a small margin of error and a high confidence level, you can obtain an accurate survey result with a small sample size. This is because the combination of these two factors compensates for the lack of precision in the sample.
To produce an accurate survey, it is vital to balance the three factors of sample size, margin of error, and confidence level; this will help you obtain a reliable and cost-effective conclusion.




